Exploring a,
b, c for a parabola.
Joshua DuMont
Parabolas with vertical lines of symmetry can be written
in the form y=ax2+bx+c , a≠0.
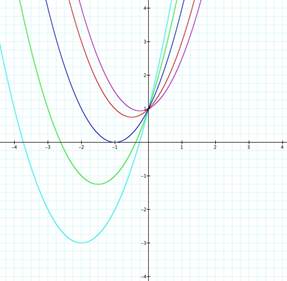
Let’s fix a=1 and b=1 in this equation and look at the
parabola for a few values of c. notice the parabola passes though the point (0,c) each time.
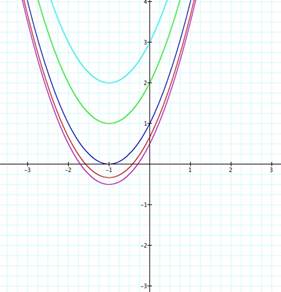
The y-intercept of a parabola in our form is the point (0,c)
We can see that this is the case by setting x=0. Then, y=a(0)2+b(0)+c=c.
From our pictures we can also guess that changing the
value of c in the equation translates the graph vertically. This is shown to be
true by taking a parabola yoriginal= ax2+bx+c1
and changing the value of c by Δc= c2-c1
The equation now reads: ynew=
ax2+bx+c2= ax2+bx+c1+ c2-c1=ax2+bx+c1+
Δc= yoriginal+
Δc. Thus each point in the original graph is
shifted up Δc units.
Parabolas are symmetric across a line called the axis of
symmetry.
If we look at the graph y= ax2+2x+1 for a
couple a values, the graphs seem to be symmetric around the line x=-1/a
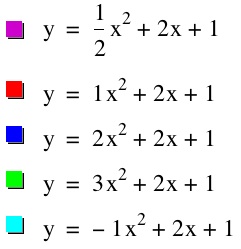
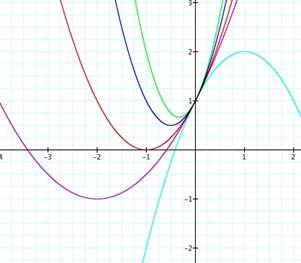
When we look at the graphs of y= x2+bx+1 for a
couple b values it looks like the graphs are symmetric around the line x=-b/2
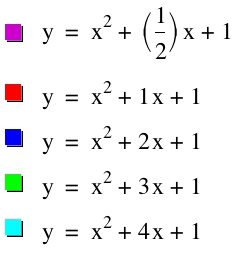
We can guess parabolas in the form y=ax2+bx+c
have an axis of symmetry: x=
.
If this is the case, then any line y=k (horizontal lines
are perpendicular to vertical ones) should intersect the parabola at two points
with x-coordinates the same distance from x=.
Setting y=k yields:
a(
=0
=0
=0
=
=
=
=
There are two x-coordinates which give a y-coordinate of
y=k and they are 
units to the right
and left of x=.
{Using a y-value of k=0 above will find the x-intercepts
of the graph at
=
= 
.
Parabolas have a highest or lowest point called a vertex.
This point can't have a reflection across the axis of symmetry or that point
would be at the same height and our choice would not be highest or lowest. This
lets us know that the vertex must be on the axis of symmetry. If we set x= then:
y= a
2+b()+c= 
+c=
The vertex of a parabola in our form is at the point:
( , ).